What’s my compression?

A while back I went through the process of calculating what the static compression ratio would be on the Cammer. I was using the cast iron block and heads but all of the internals that would eventually wind up in the aluminum engine.

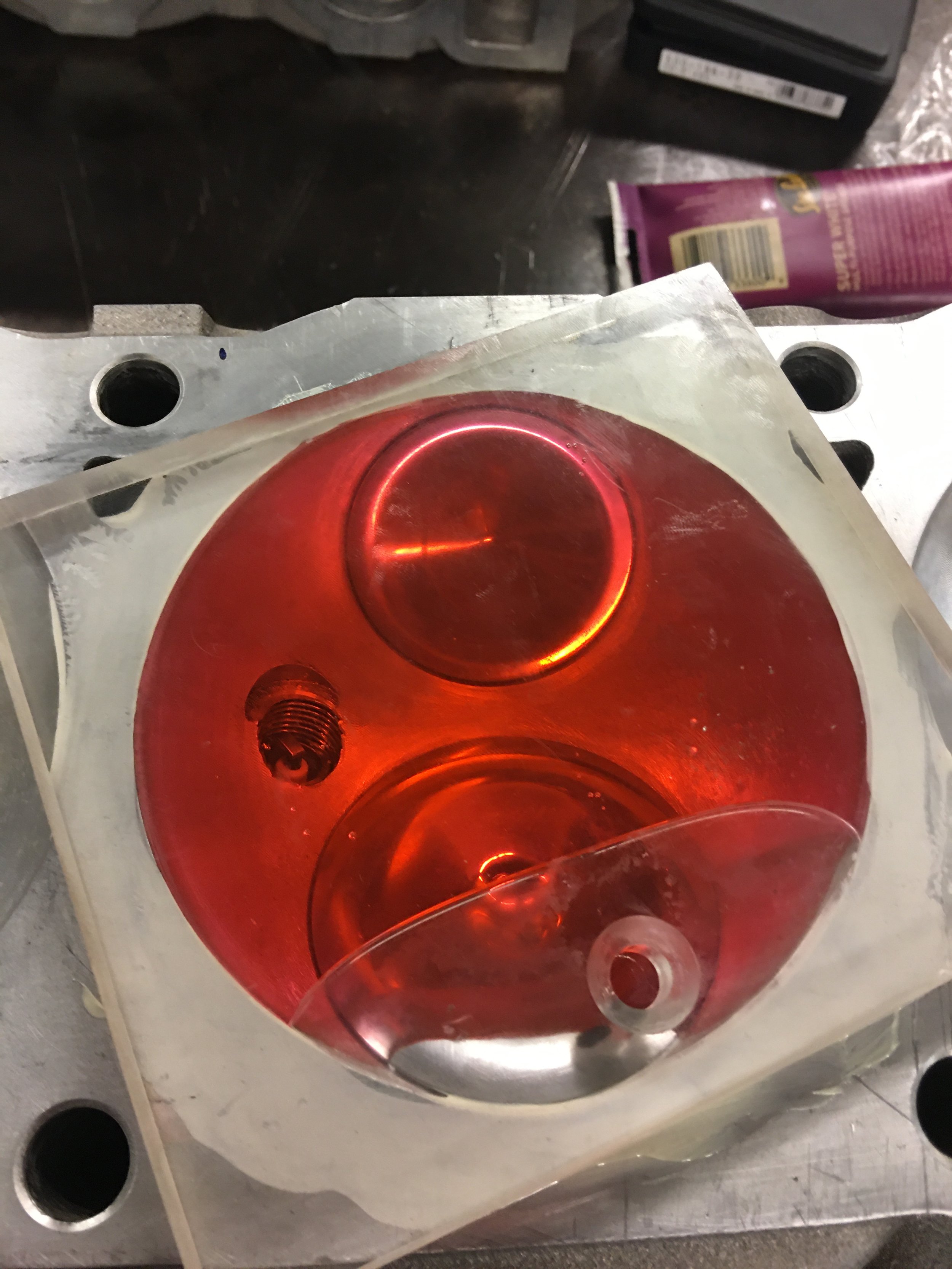
I bought a pre-drilled piece of plexiglass made just for measuring volume of the combustion chamber and piston dome.

I could have made one but for the price, it’s not really worth the effort unless you happen to have some pieces of 1/2” thick acrylic laying around.

I settled on 20 CC syringes as the means of metering the liquid which in my case was rubbing alcohol with a few drops of red food coloring added to it.

Eventually things are going to look like this:

On my first go-a-round, I did something wrong because what should have been 10.5:1 pistons had a final ratio of 10.98:1. Basically 11:1. At the time I would have sworn I did everything right and it was someone else who screwed up. I work alone by the way.

Even though it wasn’t my fault (ha), I found that I could lower the compression ratio to a more pump gas friendly 10.5:1 if I used a thicker head gasket. I did my initial calculations using a .040 thick gasket so by going to a Cometic offering of .066, I could get the CR pretty close to my target number.

10.5 is still pretty high for 91 octane gas but having aluminum heads help control detonation at these higher compression ratios.

Cometic offers gaskets that are thicker yet. their thickest is .092 and I think that might have gotten me down to 9.5 but I didn’t feel comfortable doing that.

Jump to today and I’m finally at a point in the build where I’m able to begin work on seriously putting the SOHC together and seeing as this is, literally, a new cylinder block and heads, I want fresh numbers.

I’m about to get my Nerd on here so go grab an energy drink, you’re gonna need it to stay awake.

To calculate the static compression ratio of an engine, you need an equation:

CR = (C-P+G+D+V)÷(C-P+G+D)

• C = Combustion Chamber Volume

• P = Piston Dome Volume

• G = Gasket bore volume

• D = Deck Height volume

• V = Swept Volume

Let’s get these numbers in order of the equation.

The combustion chamber volume is straight forward. Fill the combustion chamber with a thin liquid from a graduated cylinder.

As mentioned earlier, the thin liquid is rubbing alcohol with a few drops of red food coloring. The combustion chamber is sealed shut with the acrylic plate with a small fill hole drilled through it. White grease is used to hold the plate in place and seal it to the head to keep the liquid from leaking out.

Once the chamber is sealed and leak proof, it is filled with the measuring liquid with a 20 CC syringe

This gave me a reading of 126 CC, roughly. I had a low of 124 CC to a high of 127.

The next component is the piston dome volume.

The end process is very much like measuring the combustion chamber but it takes several more steps to get there.

If the components aren’t already in place like my engine is, then you have to start with building the lower end of the engine; crank, connecting rods, piston with rings. The rings don’t have to be properly gapped, the ends just can’t bind up when they’re installed into the cylinder.

With the short block assembled, or at least one cylinders worth, take the piston to the bottom of its cylinder. Measure one and a half inches down into the cylinder and smear a ring of white Lithium grease around the entire circumference.

Turn the crank to move the piston up the cylinder through the grease and bring the deck of the piston to one inch of the block deck. This is a lot easier with conventional wedge combustion chamber pistons since they actually have a deck. The Hemi style pistons have a tiny lip in line with the the piston pin, not a whole lot to square up on.

The book I was reading says to get the piston to exactly 1.00 from the top. I figure since they mention only up to 1/100, I could be a little loose with the 1/1000. I know, it’s my own screwed up logic but I just couldn’t get to 1.0000.

With the piston measuring 1 inch down in the hole, all of the excess grease must be removed from the cylinder. The excess could affect the volume count. With the cylinder cleaned up, the plastic plate is sealed to the top of the cylinder just like the combustion chamber and then the measuring can begin.

The right picture isn’t quite filled and I forgot to take a picture of the cylinder when it is completely full. That little air pocket is worth about 2 CC of fluid.

The rough number comes to 202 CC

After all of that we still only have half of the information to know what the piston dome volume is. The other half is an equation: (d÷2)(squared) X 51.48. I actually prefer another equation but it involves more steps. It’s the basic engine displacement formula: Bore x Bore x Stroke x .7854 = CI X 16.387 to convert to Cubic Centimeters. I don’t know, it’s just the way I’m wired I suppose. Anyway, the numbers I’ll type in are: 4.25 X 4.25 X 1 X .7854 = 14.19 X 16.387 = 232.47 CC. This number is the volume of a 1 inch tall cylinder that has a diameter of 4.25”

232.47 - 202 = 30.47. This is how much space the piston dome takes up.

The next value needed is to know the volume of the compressed head gasket. This is pretty simple. The gasket manufacturer will have the information needed to calculate this. In my case, the gasket bore is 4.400 inches and the thickness is .040” for this example. I’ll use the same displacement formula so once again: 4.40 x 4.40 x .040 x .7854 = .608 x 16.387 = 9.97 CC.

For a wedge engine, the piston deck height would be calculated using the displacement formula but in the case of a Hemi, there really isn’t a deck height so that will be a ZERO.

Last value for the CR formula is to compute the swept volume and again, it’s the displacement equation: 4.25 x 4.25 x 3.98 x .7854 = 65.46 x 16.387 = 925.23 CC

My initial computation was a bit off. One I took the measurements for the piston dome, a friend of mine was hanging out and we were busy talking about everything except the work at hand. Instead of the now familiar 202 CC I got, my first try netted a 181.7. This value gave me a piston dome volume over 50 CC and a CR of 13:1. A tad higher than I was expecting.

Ok, back to now. Reposting the Compression Ratio formula:

CR= (C-P+G+D+V)÷(C-P+G+D)

• C = 126

• P = 30.47

• G = 9.97

• D = 0

• V = 925.23

CR = (126-30.47+9.97+0+925.23)÷(126-30.47+9.97+0)

CR = 1030.73 ÷ 105.5

CR = 9.8

9.8:1. WTH?!?!?! The iron block and head combo gave me an 11:1 with a set up that should have been around 10.5:1? This is as bad as getting that 13.1 result. By about the 4th whack of my head against the wall, I had a thought. The combustion chamber of the Ford 427 SOHC is supposed to be 119 CC, not 126 CC. Redoing the calculation but substituting the 119 combustion chamber for the 126, I get a CR of 10.4:1.

GAWDAMMIT!!!! My combustion chambers are too big! First the oil ports in the head aren’t machined properly and now I find THIS!!!

To be honest though, this is a blessing in disguise. When I ordered my piston set, I selected 10.5: 1, down from the factory 11:1’s. Over the years I came to my senses and realized that 10.5’s weren’t great for pump gas. I looked into new lower compression pistons to get me down to 9.5:1 but that would have hit my wallet for close to 2 grand. Eeeee-yahhh, I don’t think I need to drop my compression that much. With the switch to the aluminum heads, I am told that the engine can deal with the 10.5’s on pump gas. With the unplanned for larger combustion chambers though, I have dropped my CR to under 10 and with a thicker head gasket, I can get to my sought after 9.5:1 a hell of a lot cheaper than 2 grand